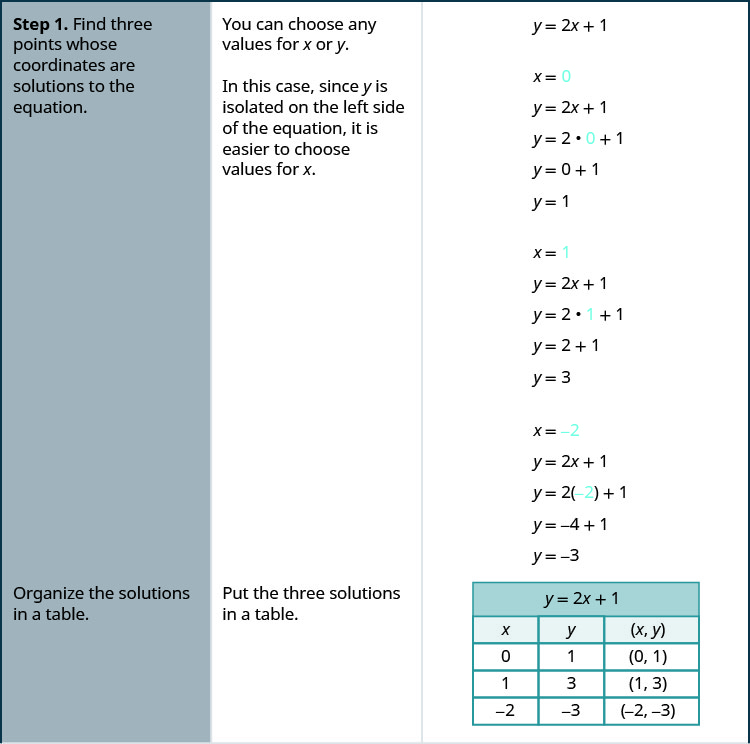
x = 4, y = 1 WARNING! i will write the first equation as $$4x^2 4y^2 8x 24y 140 = 0 \tag 1$$ form the second equation, we have $$2x = 5 3y, 4x^2 = (53y)^2 = 9y^2 30y 25 \tag 2$$ subbing $(2)$ in $(1)$ gives us $$(9y^230y25) 4y^24(53y)24y 140 = 0$$ this simplifies to $$0=13y^26y135= (y3)(13y45) $$ i hope you can take it from here Nature of Simultaneous Linear Equations The general form of a pair of linear equations in two variables is a1x1 b1y1 c1 = 0 (1) a2x2 b2y2 c2 = 0 (2) There are three conditions 1 If a1/ a2 ≠ b1/ b2, then both the equations have a

40 X Y 2 X Y 5 25 X Y 3 X Y 1
2x 3y=2 x-y/2=1/2 simultaneous equation
2x 3y=2 x-y/2=1/2 simultaneous equation-Multiply the second equation by 9 9x^2 9xy 36y^2 = 18 Substitute 3y =2x 1 9x^2 3x(2x 1) 4(2x 1)^2 = 18 9x^2 6x^2 3x 16x^2 16x 4 = 18 x^2 13x 22 = 0 (11 x)(x 2) = 0 x = 11 or x = 2 Substitute each into 3y = 2x 1The objective of simultaeous equations is to be able to work out two unknowns by using two equations in which they are both involved The first step is to label the equation xy=2 as equation 1 and 4y 2 x 2 = 11 as equation 2 Rearrange equation 1 to make one of the unknowns the subject so that we can susbititute this into the second equation leaving only one unknown



Graph Linear Equations In Two Variables Elementary Algebra
Question Solve the simultaneous equations 32x^215y^2=2112 7x^23y^2=60 What is the substitution for the quadratic formula for equation 8x^23a^2=10ax What would the problem like at the beginning x^2y^2=65 1/2xy=14 if discriminant of a complete quadratic equation is 8, what is the nature of its rootsClick here👆to get an answer to your question ️ Solve the following simultaneous equations 2x y = 2;Step by step solution of a set of 2, 3 or 4 Linear Equations using the Substitution Method 2x3y=1;y=x1 Tiger Algebra Solver
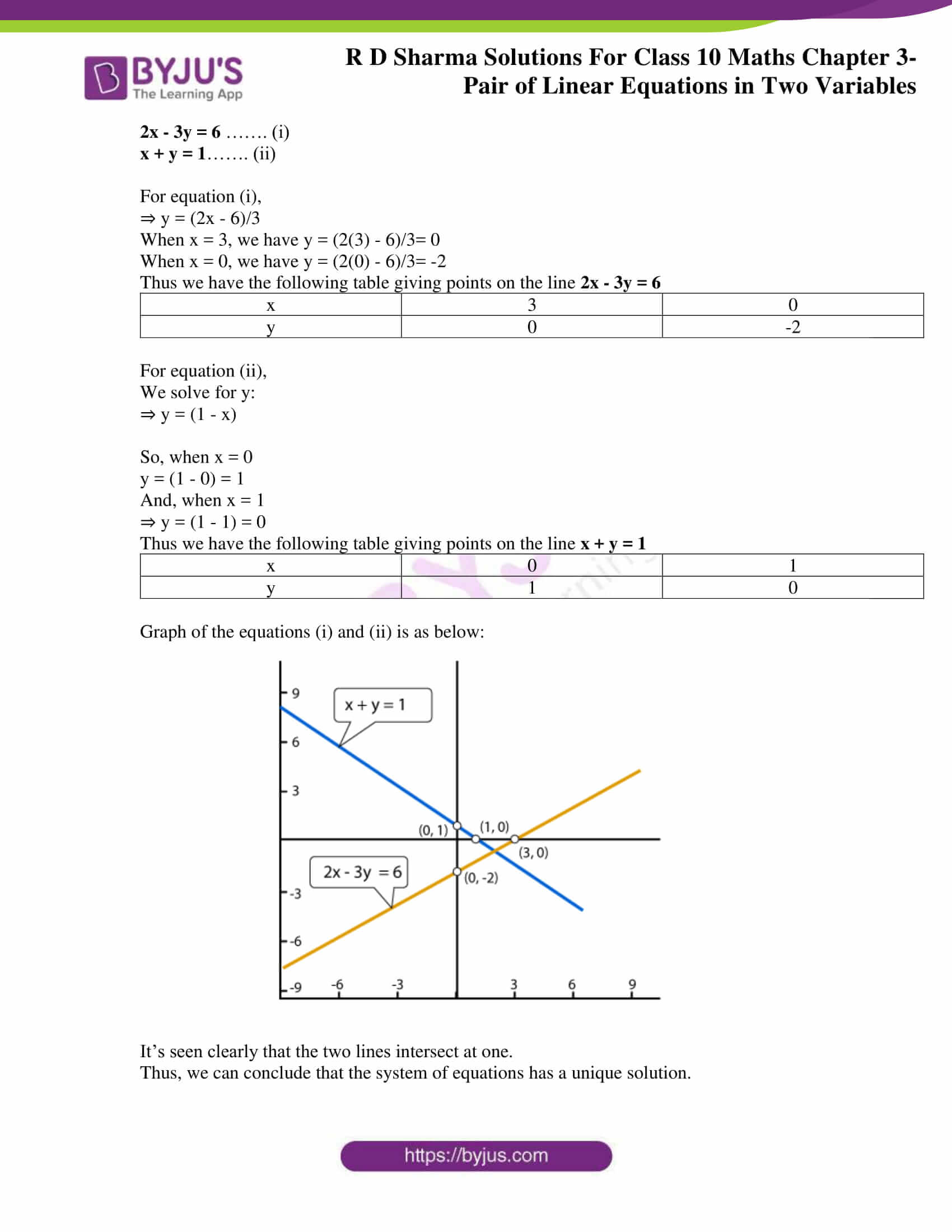
Solve the following simultaneous equations graphically 2x 3y = 4, 3y x = 4 Solve the following simultaneous equations using Cramer's rule 4 x 3y = 18, 3x – 2y = 5 Two simultaneous equations are given as 2x y = 5 and 3x y = 7 Find the value of x and y Solve the following systems of equations 2/√x 3/√y = 2 4/√x 9/√y = 1 asked Apr 26 in Linear Equations by Haifa ( 234k points) pair of linear equations in two variables
There Are Actually 4 methods of solving this We have, 2x 3y = 11(i) and, 5x 2y = 18(ii) i) Elimination Method First choose which variable you want to eliminate I'm going with y So, Multiply the eq(i) with 2 first It will turn into, 4x 6y = 22(iii) Now, Multiply the eq(ii) with 3Solve the following simultaneous equations using Cramer's rule2x 3y = 2;Quadratic Solve by Factoring;
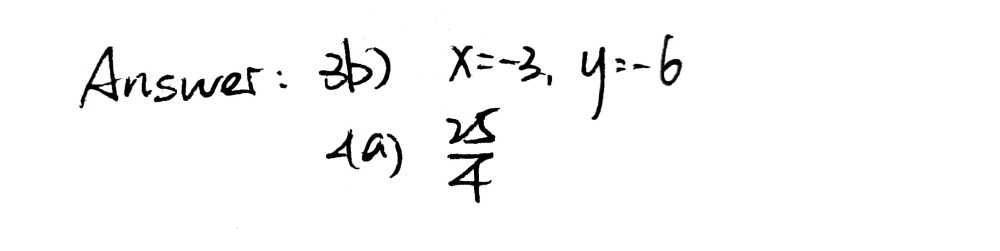



3b Find The Truth Set Of The Simultaneous Equat Gauthmath



Rd Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2
@Simon Deacon for quick reminder and GCSE maths walkthrough videosOr visit http//www3minutemathscouk for quick reminder High School GCSE mathematics vidQuadratic {1x}=10^4 \sqrt{3x}=2;Substitute x=1, y=2 in the given simultaneous equations, then (1) ab= 2 (2) a2b = 6 Subtract the second equation from the first b = 4 a = 2 substitute a=2, b=4 in the given simultaneous equations, then (3) 2x y = 4, then y = 2x 4 (4) 2x^2



Example 28 Solve By Matrix Method 3x 2y 3z 8 2x Y Z 1 Examples




Fast Track Only Answer How To Solve Linear Equations 2x 3y 2 X Y By 2 1 By 2 By Cramer S Rule Youtube
3x y = 7Yx=1,y2x=2 To solve a pair of equations using substitution, first solve one of the equations for one of the variables Then substitute the result for that variable in the other equation yx=1 Choose one of the equations and solve it for y by isolating y on the left hand side of the equal sign y=x1Now, substitute the found value x= 1 into the first equation You will get y = 2*1 1 = 2 1 = 1 Answer The solution is x= 1, y= 1 3 2y = 6x 4, y = 3x 2 In the first equation, divide both sides by 2 You will get an equivalent equation y = 3x 4 Compare it with the second equation You see that they are identical




Solving A System Of Equations Using A Matrix Precalculus Socratic
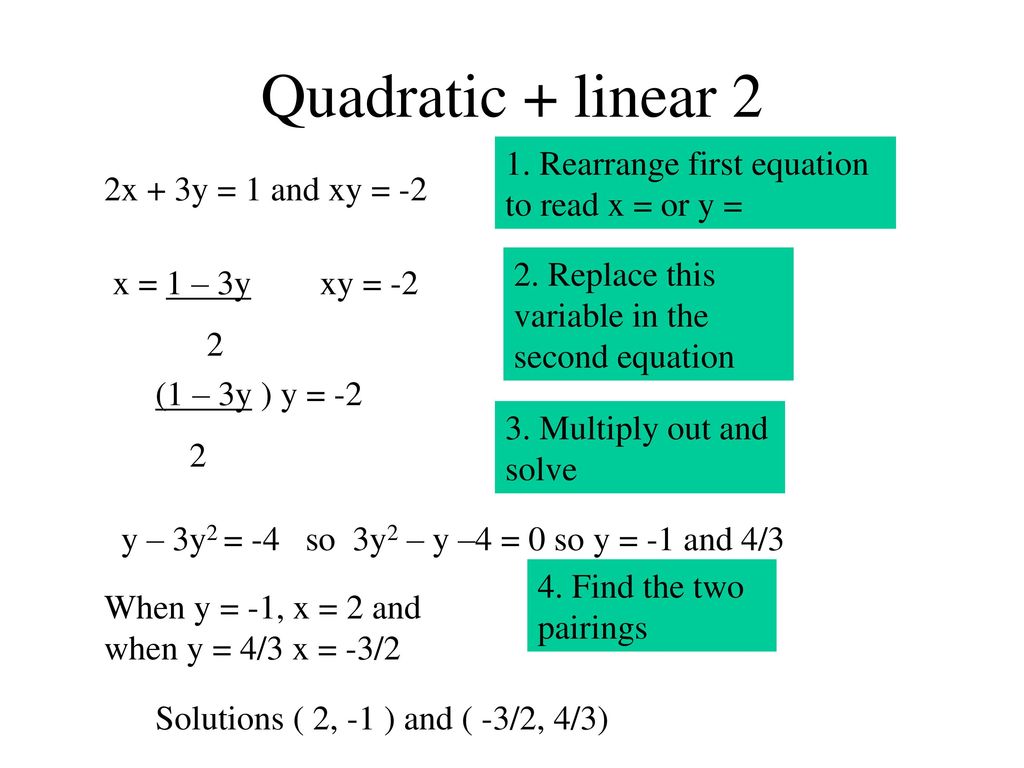



Simultaneous Equations 1 Ppt Download
2x 3 y = 7Equations Inequalities Simultaneous Equations System of Inequalities Polynomials Rationales Coordinate Geometry Complex Numbers Polar/Cartesian Functions Arithmetic & Comp Conic bernoulli y'4/x y=x^3y^2, y(2)=1 Derivatives First Derivative;Click here👆to get an answer to your question ️ Solve the following simultaneous equations 2/x 2/3y = 1/6;




Solving Linear Systems



Www Sgasd Org Site Handlers Filedownload Ashx Moduleinstanceid 9221 Dataid Filename Answer key Pdf
Step by step solution of a set of 2, 3 or 4 Linear Equations using the Substitution Method 2x3y=2/3;3x4y=18 Tiger Algebra SolverAs both equations are equal to y, this also means they are equal to each other So firstly, substitute the simpler equation which is y=2x2 into the second equation giving 2x2 = x^2 1 Rearrangement of this gives x^2 2x 3 = 0 Using quadratic equation theory, this then becomes (x 3)(x 1System of Equations Calculator y= x2,2x3y=1 Equations Basic (Linear) Solve For;




Simplify 11 2x 2y 9 4x Y 2 1 4x Y 1 14 Y 2x 1 15 Y X 2 1 2x Y




Exercise 1 2 Cramer S Rule Problem Questions With Answer Solution
Use the substitution method to solve the system of equations 2x 4y = 16 6x 3y = 18 Equation 1 is in the correct ax by format Equation 2 is in the correct ax by format Rearrange Equation 2 to solve for x 6x 3y = 18 Add 3y to both sides to isolate x 6x 3y 3y = 18 3y 6x = 18 3y Now divide both sides by 6Solve the Following Simultaneous Equations Using Cramer'S RuleX 2y = –1 ;Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more




4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula



Solve The Following Simultaneous Equations Using Cramer S Rule I 6x 4y 12 8x 3y 2 Ii 4m 6n 54 3m 2n 28 Sarthaks Econnect Largest Online Education Community
Solve the simultaneous equations 2x^23y^2=6 and 3x^22y^2=35 Latest Problem Solving in Fundamentals in Algebra More Questions in Fundamentals in Algebra Online Questions and Answers in Fundamentals in Algebra Series2x – 3y = 12 0 Maharashtra State Board SSC (English Medium) 10th Standard Board ExamX y2=12 Maharashtra State Board SSC (English Medium) 10th Standard Board Exam Question Papers 238 Textbook Solutions Online Tests 39 Important



Files Schudio Com Brgs Files Edexcel Transistion From Gcse To A Level Files 1 Pdf
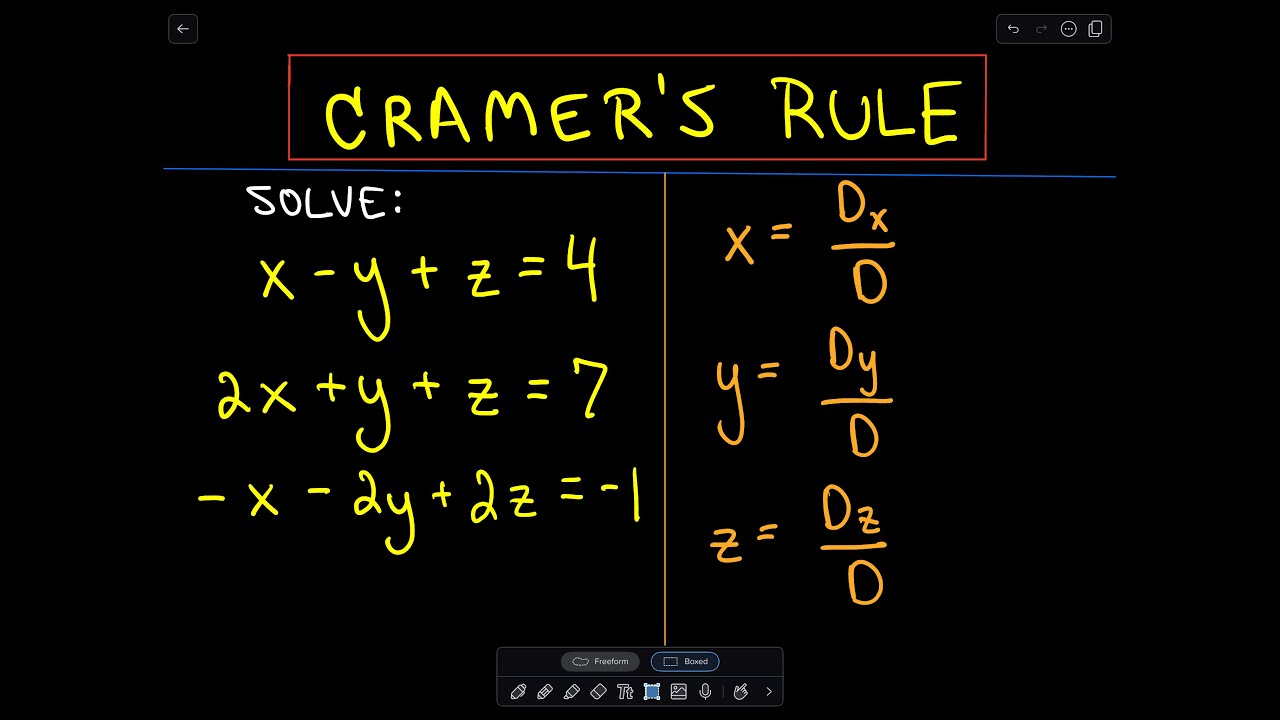



Cramer S Rule To Solve A System Of 3 Linear Equations Example 2 Youtube
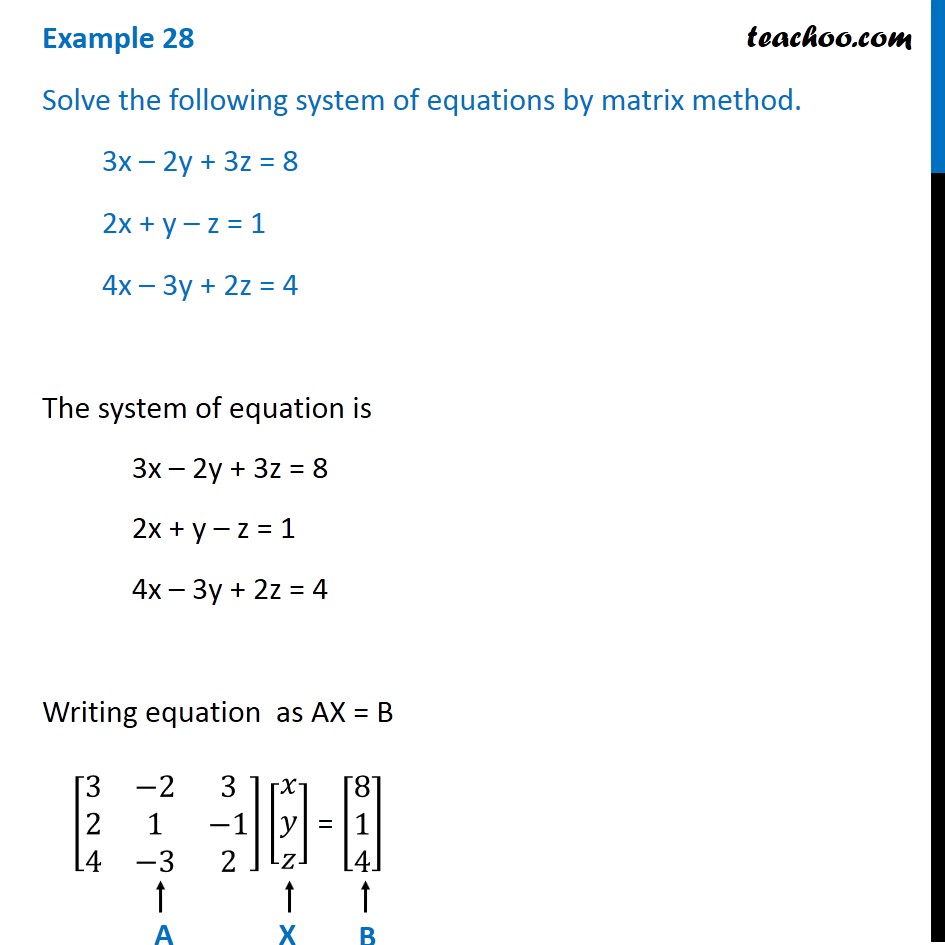
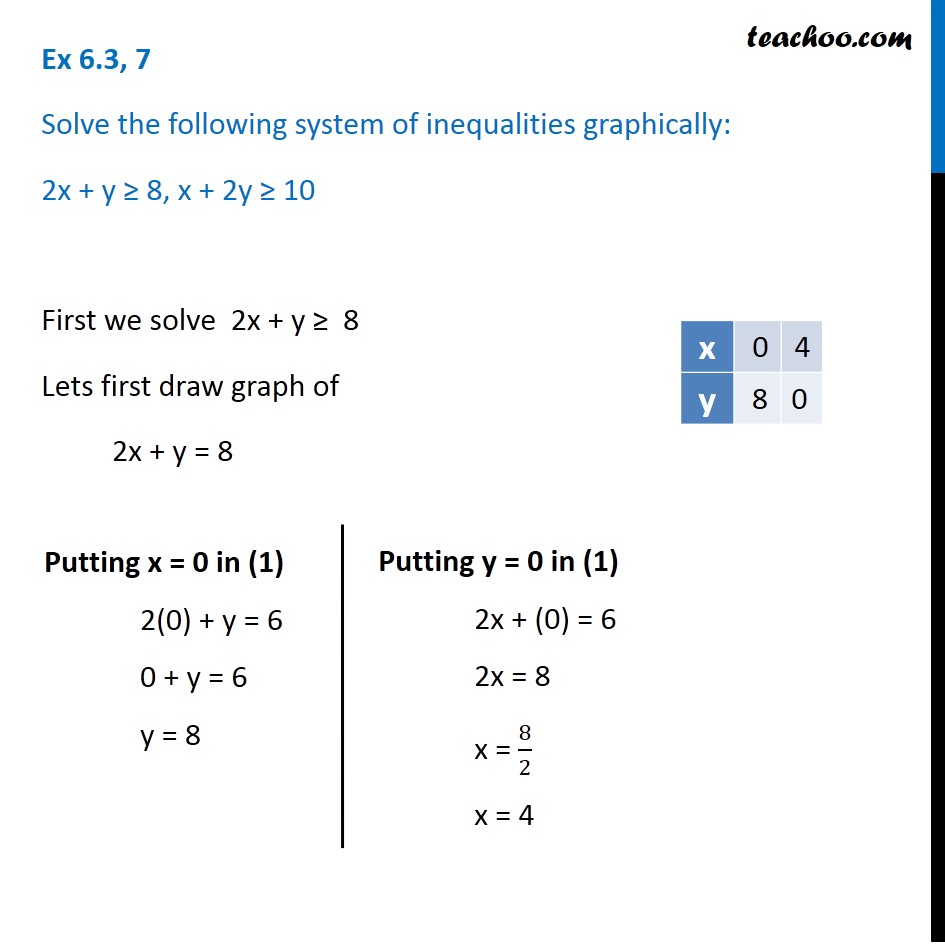
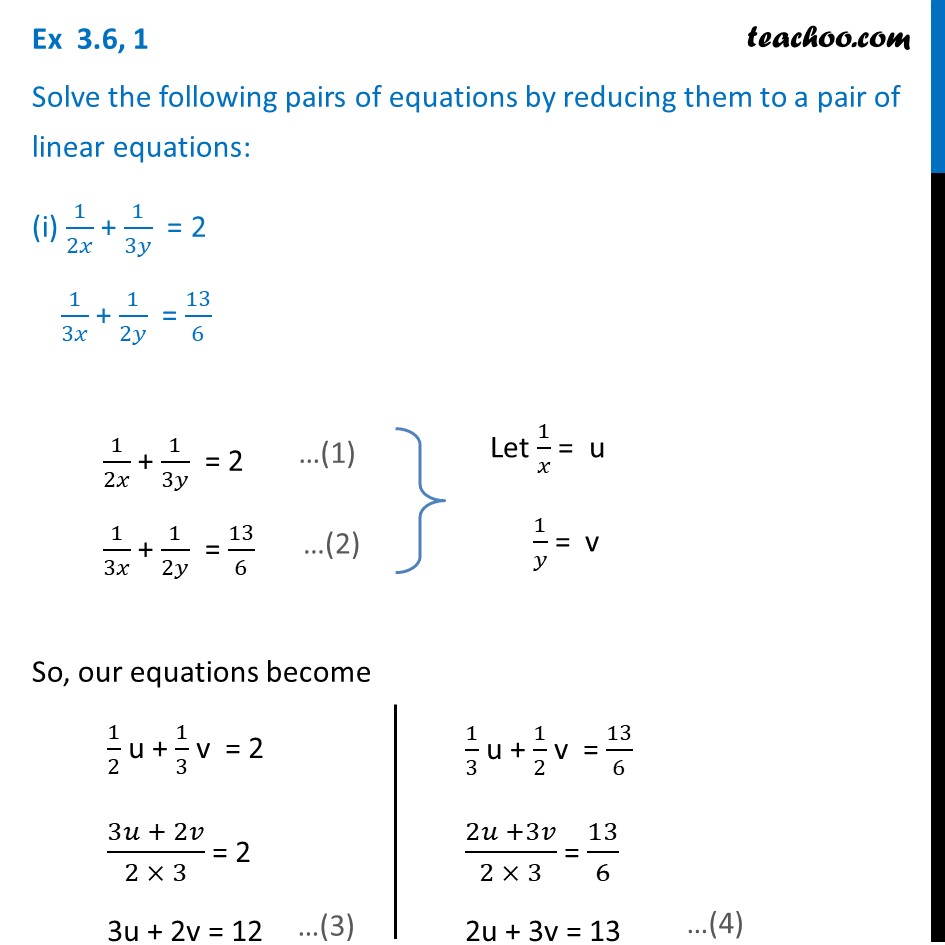
Specify Method (new) Chain Rule; Transcript Ex 36, 1 Solve the following pairs of equations by reducing them to a pair of linear equations (i) 1/2𝑥 1/3𝑦 = 2 1/3𝑥 1/2𝑦 = 13/6 1/2𝑥 1/3𝑦 = 2 1/3𝑥 1/2𝑦 = 13/6 Let 1/𝑥 = u 1/𝑦 = v So, our equations become 1/2 u 1/3 v = 2 (3𝑢 2𝑣)/(2 × 3) = 2 3u 2v = 12 1/3 u 1/2 v = 13/6 (2𝑢 3𝑣)/(2 × 3) = 13/6 2u 3v = 13 Our equations2x 3y = 14 eqn(1) 3x 2y = 6 eqn(2) There are two methods in solving simultaneous equation 1) Substitution method 2) Elimination method
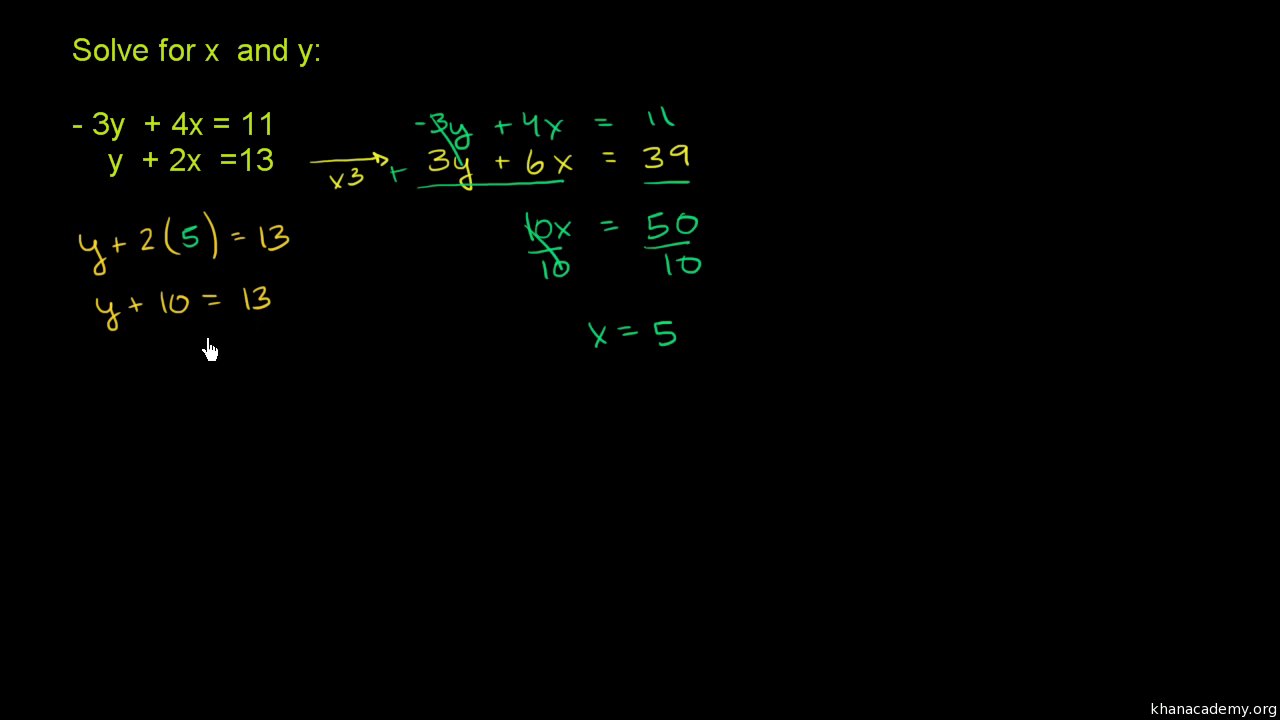



Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy




Tim Kerins Leaving Certificate Honours Maths Algebra Tim Kerins The Date Pdf Free Download
Xy/2=1/2 solve simultaneous equation Ask for details ;Simultaneous Equations Calculator Solving simultaneous equations is one small algebra step further on from simple equations Symbolab mathX^2 x^{\msquare} \log_{\msquare} \sqrt



If X Y 3 X Y 1 Then What Is X Y Quora



Simultaneous Equations Gmat Math Study Guide
Equations Inequalities Simultaneous Equations System of Inequalities Polynomials Rationales Coordinate Geometry Complex Numbers Polar/Cartesian Functions Arithmetic & Comp Conic Sections Trigonometry x^22xy3y^2=3, x^23xy2y^2=4 Equations Basic (Linear) Solve For;Solve the following pair of simultaneous equations 2x3y=13 3xy=3 math solve for x and y , the simultaneous equations 4^x3 = 32(2^xy) and 9^x 3^y = 10 Math 3 Solve the simultaneous equations x² y² = 34 x – y = 2 Maths solve in simultaneous method a2b=13 2a3b=5 Mathematics Comparing the given equations with a 1 x b 1 y = C 1 and a 2 x b 2 y = c 2, we get a 1 = 1, b 1 = 2, c 1 = 1 and a 2 = 2, b 2 = 3, c 2 = 12 ∴ (x, y) = (3, 2) is the solution of the given simultaneous equations iv The given simultaneous equations are 6x – 4y = 12 ∴ 3x – 2y = 6 (i) Dividing both sides by 2 8x – 3y = 2



Www Omtexclasses Com 12 Problem Set 1 Linear Equations In Two Variables Class 10th Mathematics Part 1 Mhb Solution Html




Chapter 13 Solving Simultaneous Equations
Solve the following simultaneous using Cramer's rule2x 3y = 2, x− 13 Q3 (6)Learn more about Linear Equation with t Solve the following simultaneous using Cramer's rule2x 3y = 2X3y = 2 2x3y = 6 now subtract the 1st from the 2nd and you have x = 4 Then plug that into either equation to find y 43y = 2 y = 2/3 final step check those values in each of the original equations to make sure you have not made a mistake somewhere$2x = 1 3y$ $3y^2 x^2 = 2$ Solve these simultaneous equations Stack Exchange Network Stack Exchange network consists of 178 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn,




X 3 5y 13 2x Y 2 19 X 3 5y 13 2x Y 2 19 Josspix0sfi




Graph Linear Equations In Two Variables Elementary Algebra
Simultaneous Equations xy=4 and x^2y^2=40 This question consists of a linear equation and a quadratic equation , which you have to solve using the simultaneous method There will be two sets of solutions for both x and y, because if you were draw the graphs of both equations, the straightline graph will intersect the quadratic curve in two places giving two solutions Find an answer to your question simultaneous equation using cramer's rule 2x3y=2;xy/2=1/2The simultanous equation calculator helps you find the value of unknown varriables of a system of linear, quadratic, or nonlinear equations for 2, 3,4 or 5 unknowns A system of 3 linear equations with 3 unknowns x,y,z is a classic example This solve linear equation solver 3 unknowns helps you solve such systems systematically




Systems Of Equations With Elimination X 2y 6 4x 2y 14 Video Khan Academy
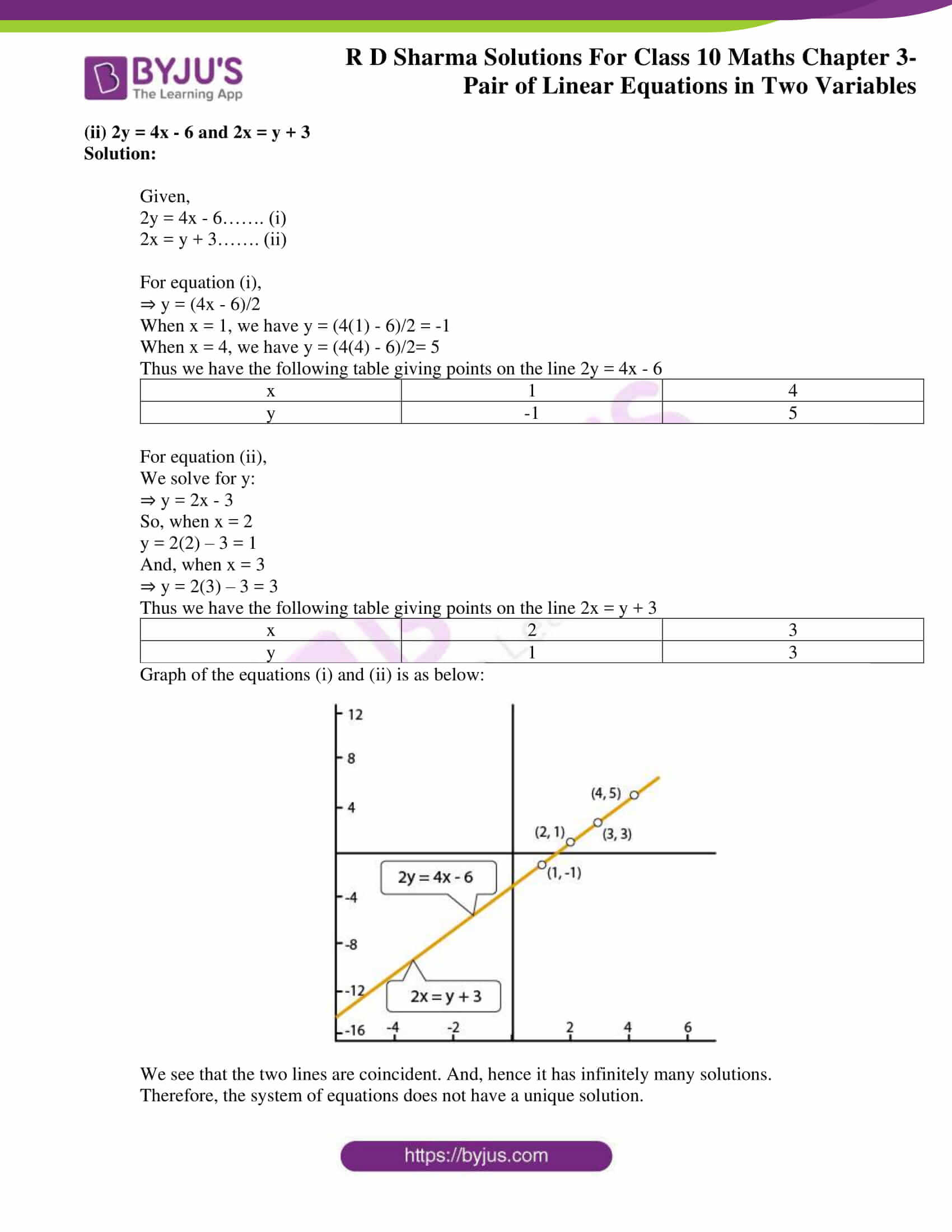



Rd Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2
Thus, if x, y are such that x 2 3 y 2 = 1 then x 2 y 2 ≤ 1 Show that there are no rational solutions on the ellipse 2x^2 3y^2 = 1 Show that there are no rational solutions on the ellipse 2 x 2 3 y 2 = 1Follow Report by Vishwajeet17 Log in to add a comment3/x 2/y = 0




Solve Simultenous Equations Using Cramer S Rule 2x 3y 2 X Y 2 1 2 Brainly In




If 2x 3y 14 And 2x 3y 2 Find The Value Of Xy
Math, 0810, jack 2x3y=2 ;Simultaneous equations are a pair of equations where there is more than one unknown value There are several methods you can use to solve them 59K people helped x—2 equations combined 2 (2x 3y) 3 (5x 2y) = 2 (11) 3 (18) 4x 6y 15x 6y = 22 54 19x = 76 x = 4 y—1st equation, substitute x 2 (4) 3y = 11 8 3y = 11 3y = 3 y = 1 Answer x = 4, y = 1 Proof—2nd equation 5 (4) 2 ( 1) = 18 2 = 18 18 = 18 heart outlined Thanks 0 star outlined star



X 2 Y 2 0 Graph



Www Katemarshallmaths Com Uploads 1 8 8 2 Simultaneous Equations Practice Test14 Pdf
Click here👆to get an answer to your question ️ Solve the following simultaneous equations x y = 11 ; Solve graphically the simultaneous equations given below Take the scale as 2 cm = 1 unit on both the axes x – 2y – 4 = 0 2x y = 3 Answer 2 Question 3 Use graph paper for this question Draw the graph of 2x – y – 1 = 0 and 2x y = 9 on the same axes Use 2 cm = 1 unit on both axes and plot only 3 points per lineSimple and best practice solution for 2x3y=3 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand,
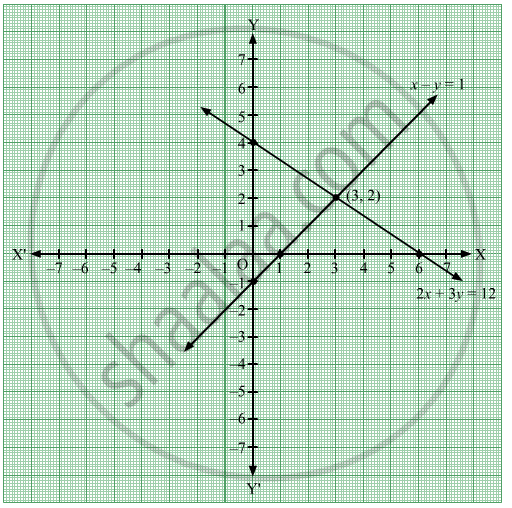



Solve The Following Simultaneous Equation Graphically 2x 3y 12 X Y 1 Algebra Shaalaa Com
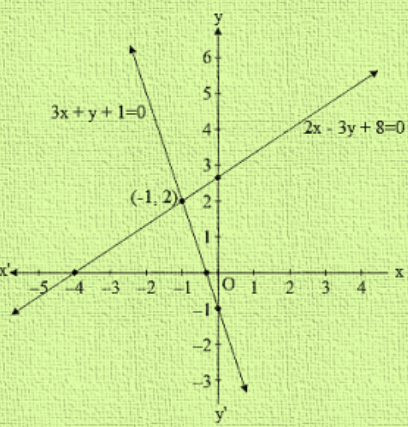



R D Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2
Solve by Substitution 2x3y=1 y=x1 Replace all occurrences of with in each equation Tap for more steps Replace all Solve for in the first equation Tap for more steps Move all terms not containing to the right side of the equation Tap for more steps Subtract from both sides of the equation Subtract from Multiply each term The two solutions are (7,5) and (5,1) To solve the system of equations {(x2y=3,qquad(1)),(x^2y^2=24,qquad(2))} We must realize that there may be two solutions, since equation (2) is a hyperbola, and hyperbola may cross a linear equation at two points First, solve for x in equation (1) x2y=3 x=32y Plug this into equation (2) x^2y^2=24 (32y)^2y^2=24 912y4y^2y^2=24 912y3y^2Question Solve the simultaneous equation y2x1=0 and 4x^23y^22xy=7 Related Answer More Related Question & AnswersMore Related Question & Answers



Www Topperlearning Com R S Aggarwal And V Aggarwal Solutions Cbse Class 10 Mathematics R S Aggarwal And V Aggarwal Mathematics X Linear Equations In Two Variables



Search Q 2x 3y 3d2 Graph Tbm Isch
Solve each of the following systems of simultaneous linear equations by the method of substitution (i) 3x 2y = 11, 2x 3y = 4 asked in Linear Equations in Two Variables by HarshKumar ( 327k points)




Graph Graph Inequalities With Step By Step Math Problem Solver




Graph Graph Inequalities With Step By Step Math Problem Solver
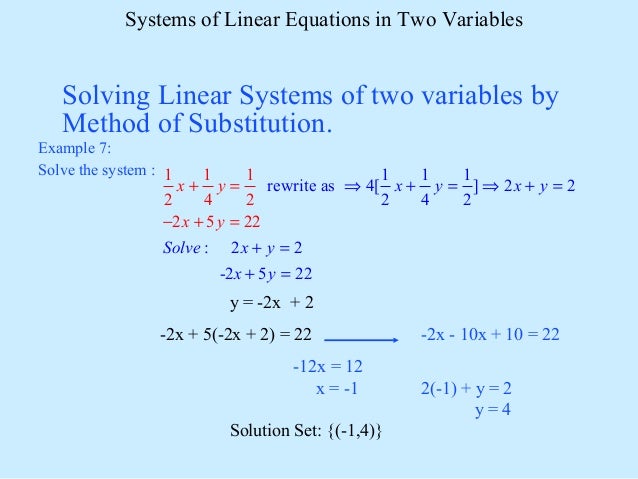



Solving Systems Of Linear Equations




2x 3y 2 X Y 2 1 2 Using Cramers Rule Brainly In
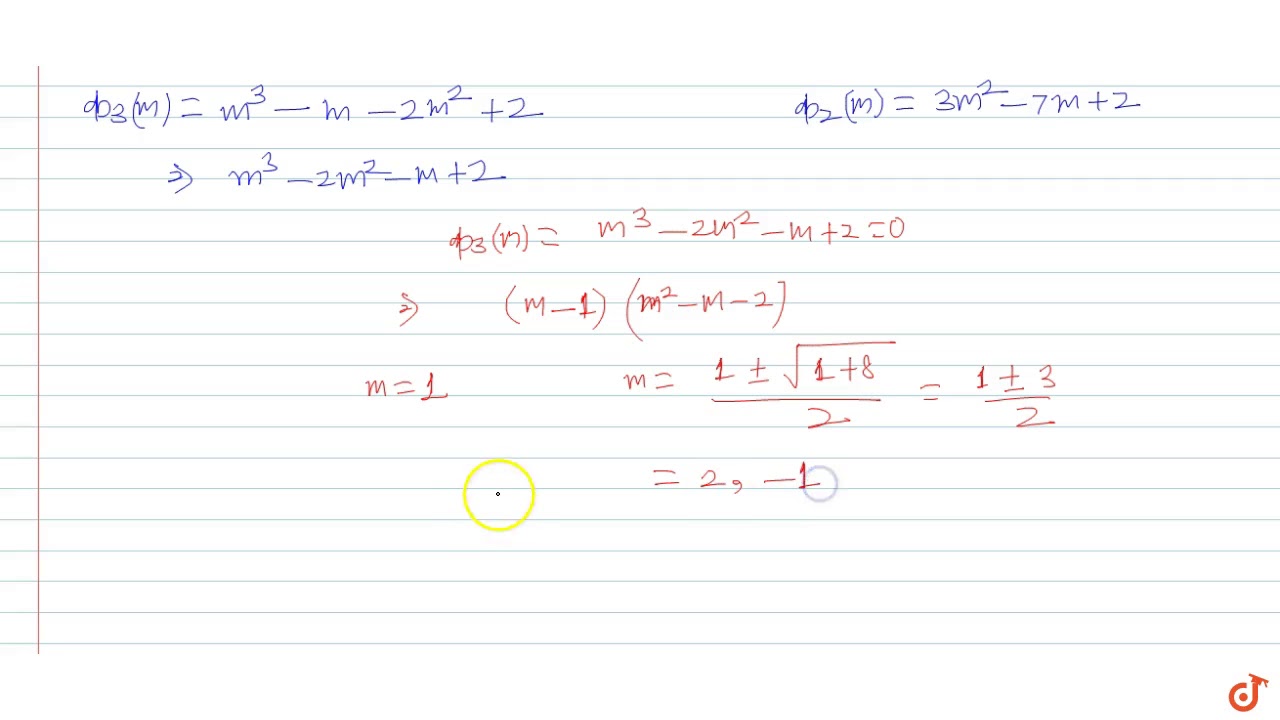



7x 3y 1 0



The Substitution Method
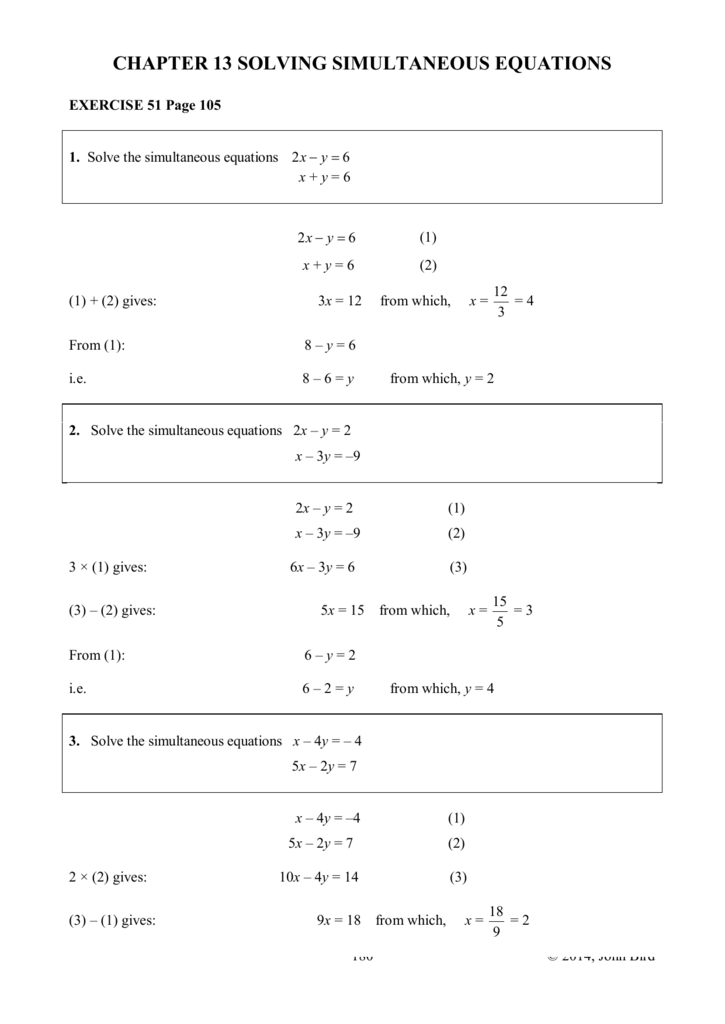



Chapter 13 Solving Simultaneous Equations




4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula



Graphs And Solutions To Systems Of Linear Equations Beginning Algebra




40 X Y 2 X Y 5 25 X Y 3 X Y 1




1 2 1 2 1 2 1 Using Elementury Tbos Ou Tiu Hence Solve The System Of Equations 2y Z 90 2y 22 9 2x Y 2 2 Consider Dx 3b Where A Il 21 U 1a I 1 2 2 1 4 1 1 2 3 10 8 0 Exist Consider I S
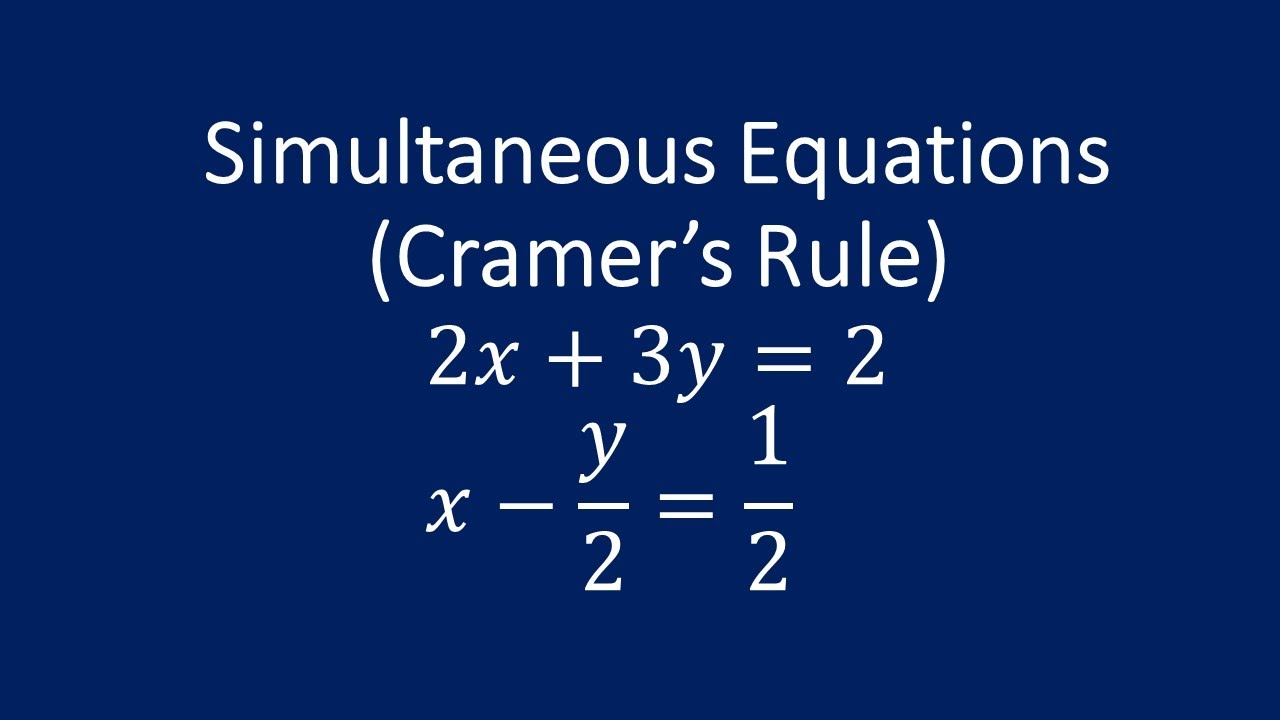



Simultaneous Equation 4 Youtube



Q Tbn And9gctlssrhblrcme30srq3fcq6nav2j5koc70zetfuvq4sst Grxag Usqp Cau
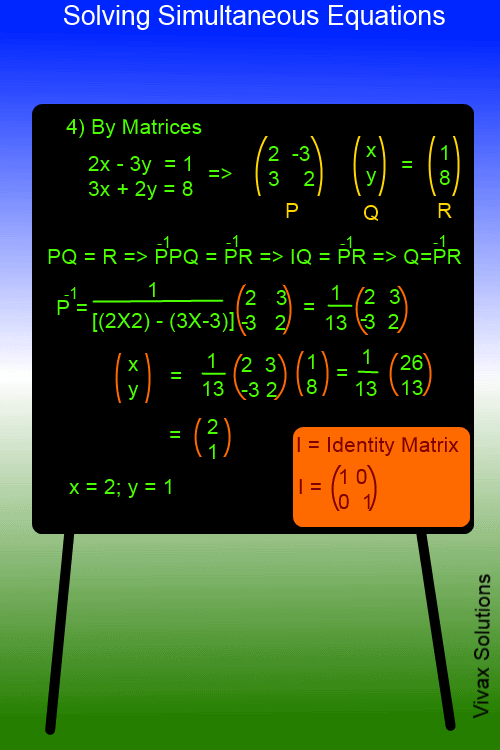



Solving Simultaneous Equations Elimination Substitution Graphical Matrix Methods Maths Tutorials Vivax Solutions




Omtex Classes 3x Y 2 2x Y 3 Solve The Following Simultaneous Equations Graphically
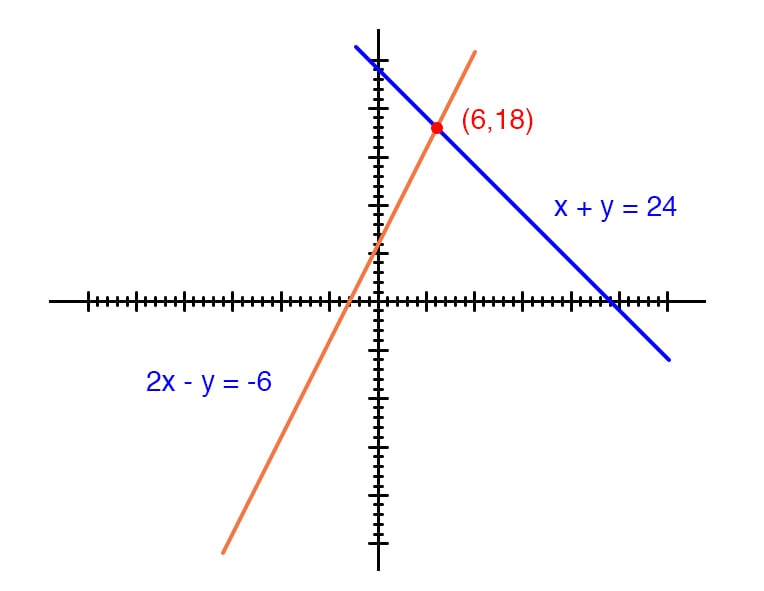



Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook
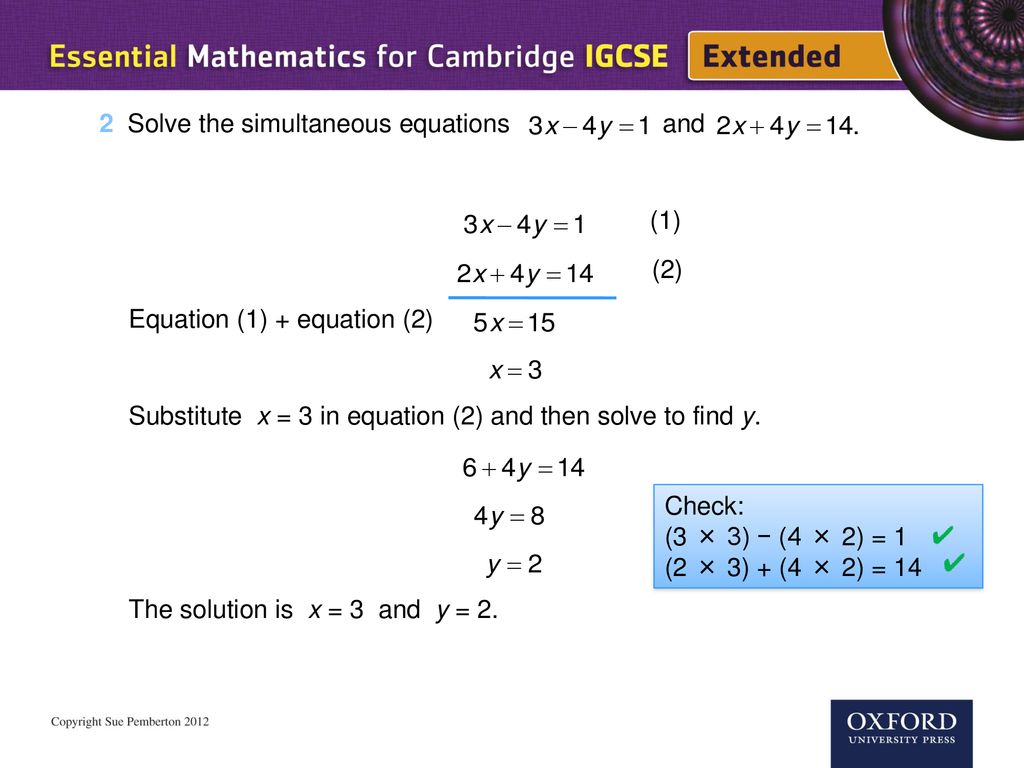



Simultaneous Equations 1 Ppt Download




Solve Systems Of Linear Equations With Two Variables Intermediate Algebra
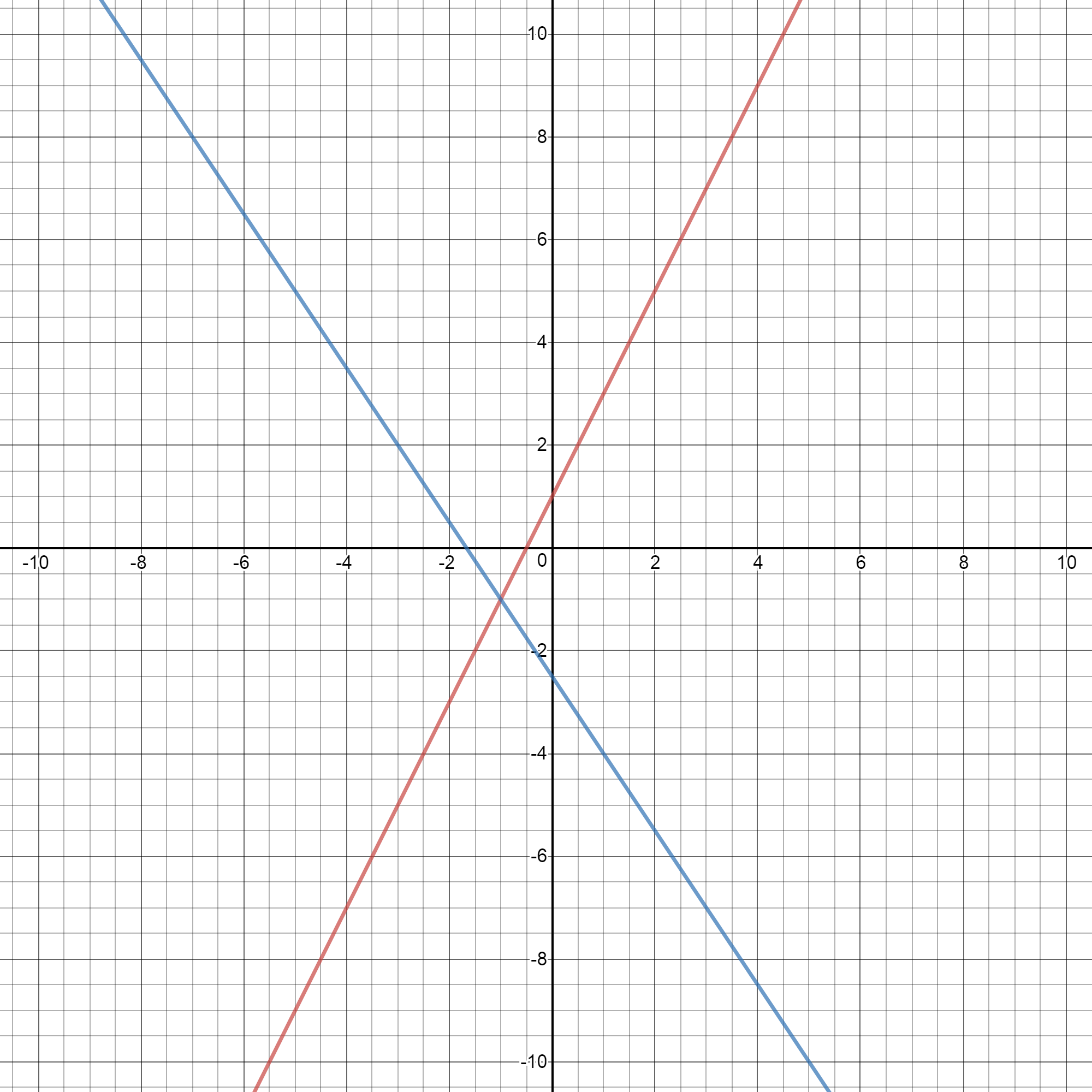



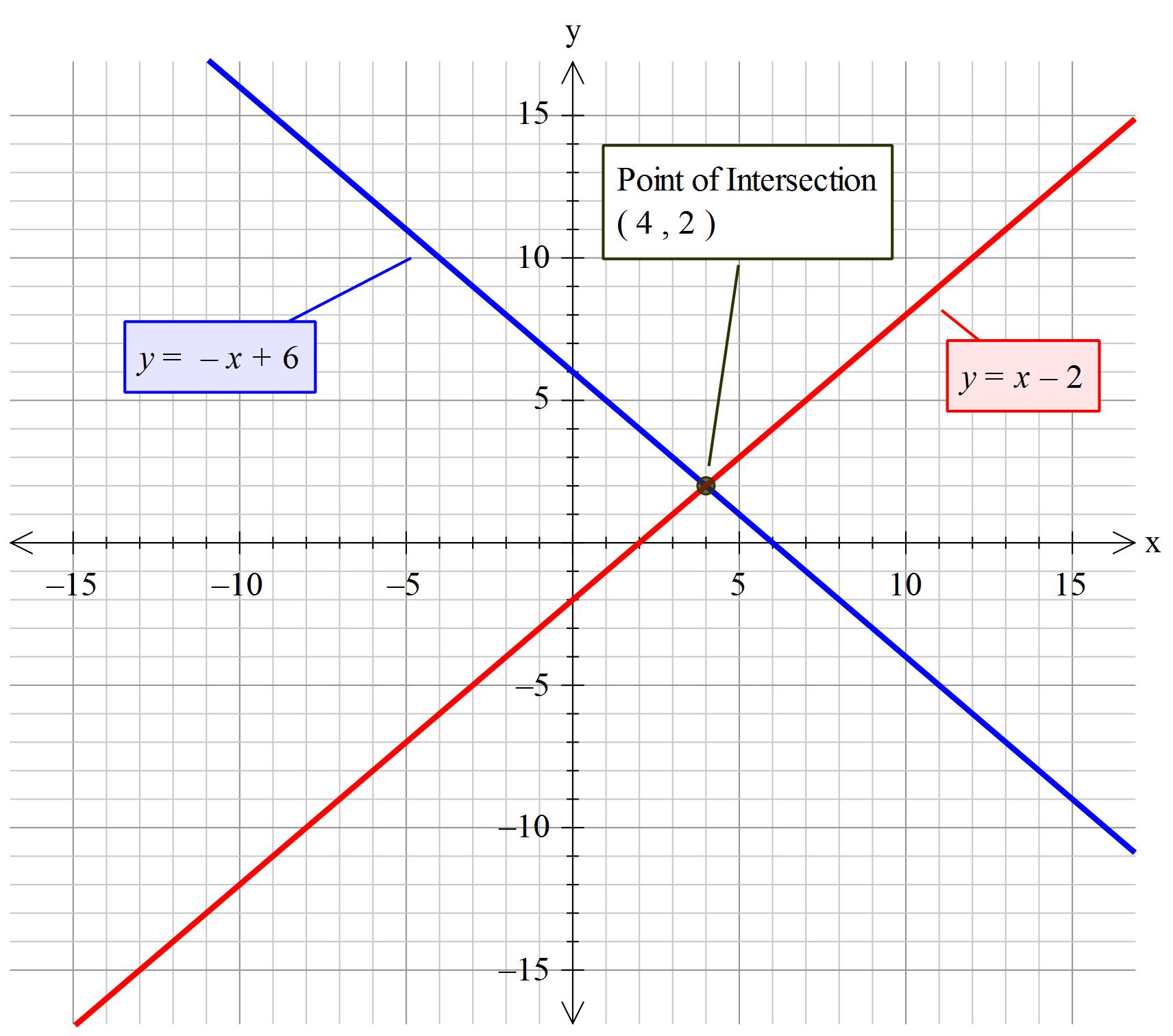
How To Solve The System Of Equations 2x Y 1 And 3x 2y 5 By Graphing Socratic



Part 8 Simultaneous Equations Beginner S Guide To Year 9 Maths



How To Solve The Simultaneous Equation Xy 2 2log X 1 Log Y Quora




2x 3y 2 X Y 2 1 2 Practice Set 1 3 Crammers Method क र मरच पद धत Crammers Rule Grade 10 Youtube




2 X 2 3y 1 6 And 3 X 2 Y 0 Problem Set 1 Q6 1 Of Linear Equation In Two Variables Youtube



Www Waynesville K12 Mo Us Cms Lib07 Mo Centricity Domain 603 A1 c6 l2 solution key worked out Pdf




Factoring Forms 1 Introduction X Y 2 X 2 2y 2 4y 2 1 Eq1 2 1 Y X Pdf Free Download



Http Portal Unimap Edu My Portal Page Portal30 Lecture notes Imk Semester 2 sidang akademik 0910 Eqt102 Persamaan pembezaan Pdf



Link Springer Com Content Pdf m 3a978 1 349 3 2f1 Pdf




Solutions To Implicit Differentiation Problems



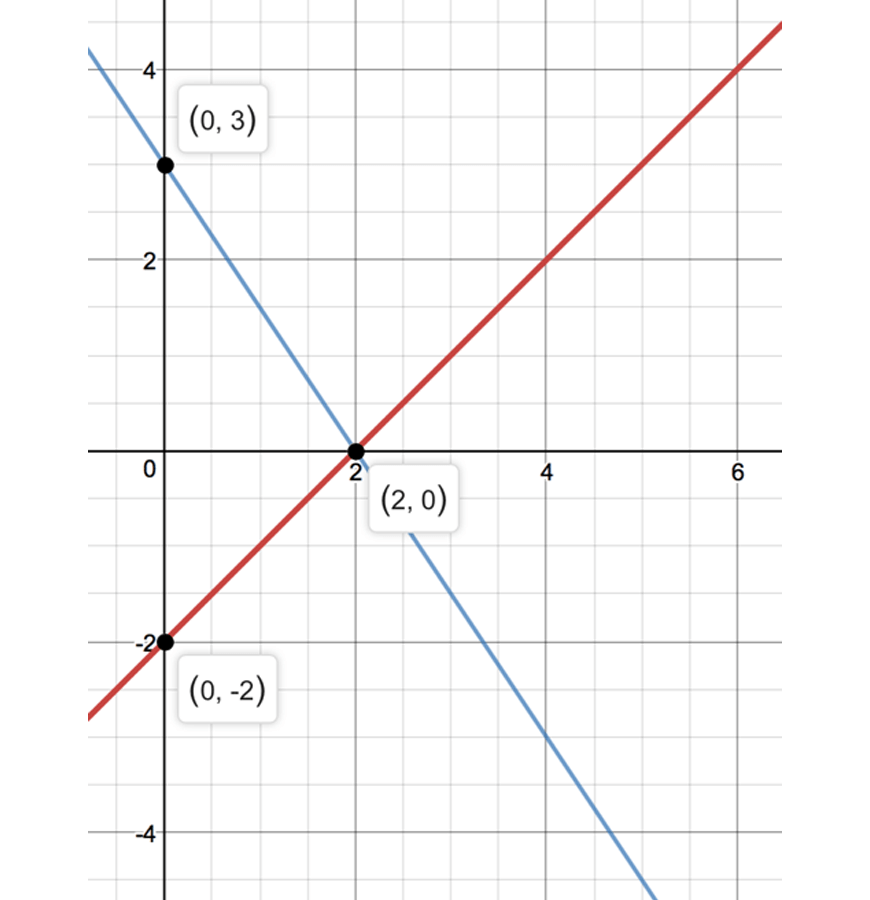
How Do You Solve The System X Y 6 And X Y 2 By Graphing Socratic
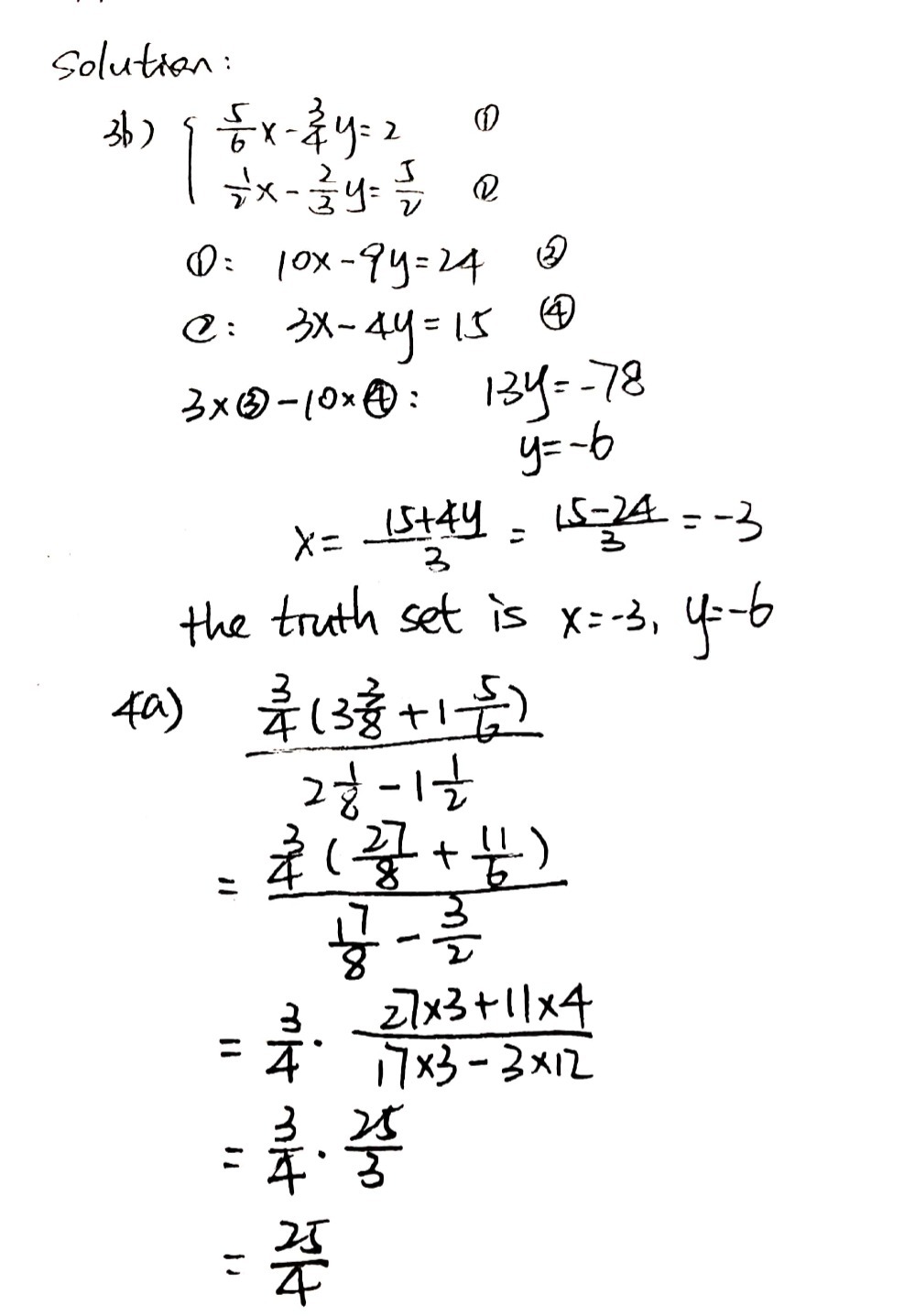



3b Find The Truth Set Of The Simultaneous Equat Gauthmath




4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula



How To Solve The Simultaneous Equation Xy 2 2log X 1 Log Y Quora




70以上 Y2x2 Z2 ニスヌーピー 壁紙



Maths Guru Please Help Me Solve This Simultaneous Equations For My Young Photo Education 2 Nigeria



Link Springer Com Content Pdf m 3a978 1 349 3 2f1 Pdf




7x 3y 1 0
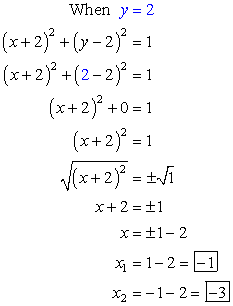



Systems Of Non Linear Equations Chilimath




Solve The Following Simultaneous Equations Using Cramer S Rule 2x 3y 2 X Y 2 1 3 Brainly In




Exercise 1 2 Cramer S Rule Problem Questions With Answer Solution



How To Solve This Simultaneous Linear Equation 3x 4y 7 0 2x Y 2 0 Quora




最も欲しかった 2x 3y 2 X Y 2 1 2 6 2x 3y 2 X Y 2 1 2



Azrael Digipen Edu Mat180 Newtonsmethod Pdf




Mathematics Ii Tranquileducation



Www Ualberta Ca Csproat Homework Math 334 Assignment solutions Assignment 2 solutions Pdf



Maths Guru Please Help Me Solve This Simultaneous Equations For My Young Photo Education 2 Nigeria




Solving Linear Systems



2fv5d843v9w22sxtto1ibxtu Wpengine Netdna Ssl Com Wp Content Uploads 15 12 Algebra H Simultaneous Equations V2 Solutions V3 Pdf



Systems Of Linear Equations



Solve The Following Simultaneous Equations Using Cramer S Rule I 6x 4y 12 8x 3y 2 Ii 4m 6n 54 3m 2n 28 Sarthaks Econnect Largest Online Education Community




Solve The Following Simultaneous Equation Using Cramer S Rule X 2y 1 2x 3y 12
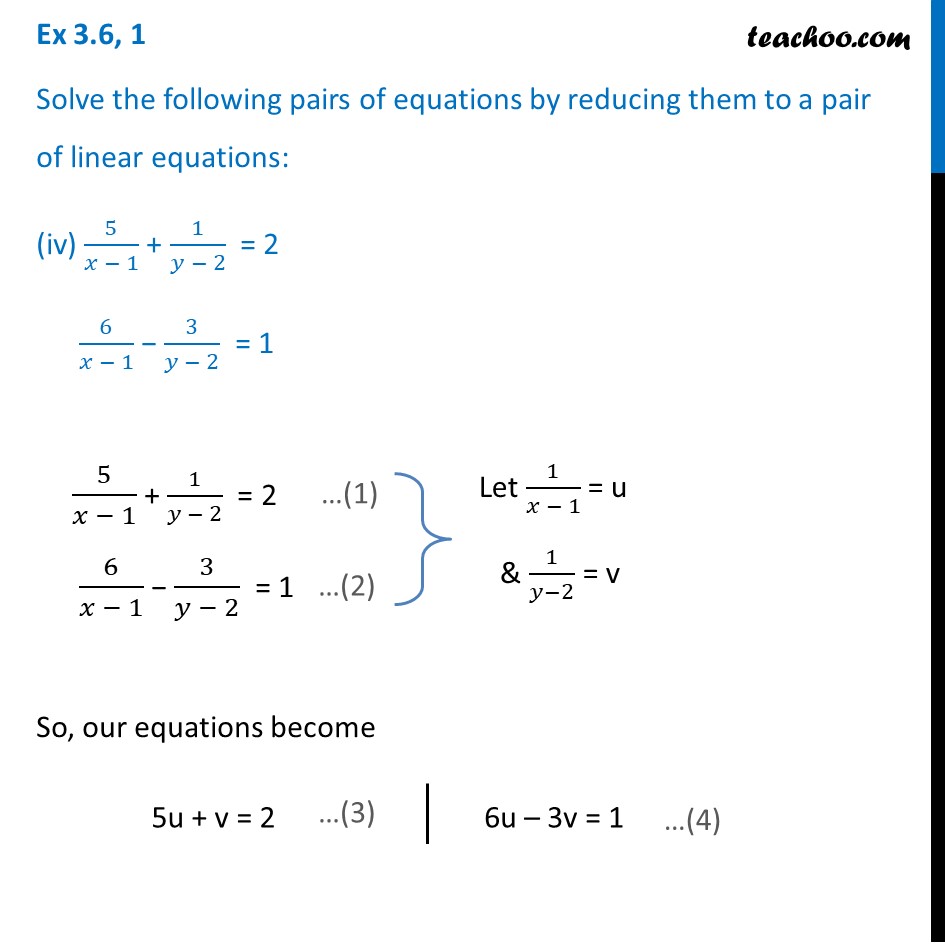



Ex 3 6 1 Iii Iv 4 X 3y 14 3 X 4y 23 Ex 3 6




2x 3y 2 X Y 2 1 2 Using Cramer S Rule Brainly In




Rewrite The Slope Intercept Equation Of The Line Y 1 3 X 2 In



1



Q Tbn And9gcqx Bkrxl1qn Pz27cang Z2q8 Vuh Zl2b3uxknbehnuvf5c8 Usqp Cau




最も欲しかった 2x 3y 2 X Y 2 1 2 6 2x 3y 2 X Y 2 1 2



Solve The Following Systems Of Equations 3 X Y 2 X Y 2 9 X Y 4 X Y 1 Sarthaks Econnect Largest Online Education Community
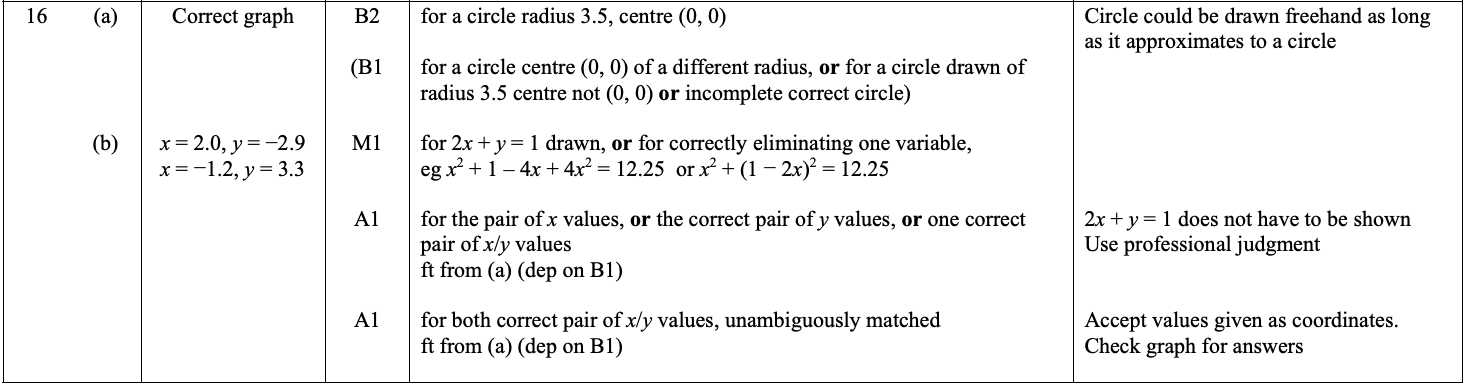



Q16 Answers Paper 2 June 18 Edexcel Gcse Maths Higher Elevise




2x 3y 2 X Y 2 1 2 Solve The Following Simultaneous Equations Using Cramer S Rule Brainly In
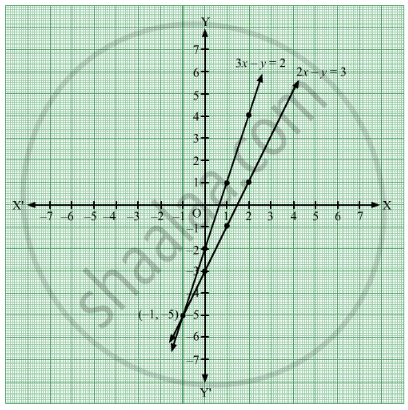



Solve The Following Simultaneous Equations Graphically 3x Y 2 2x Y 3 Algebra Shaalaa Com
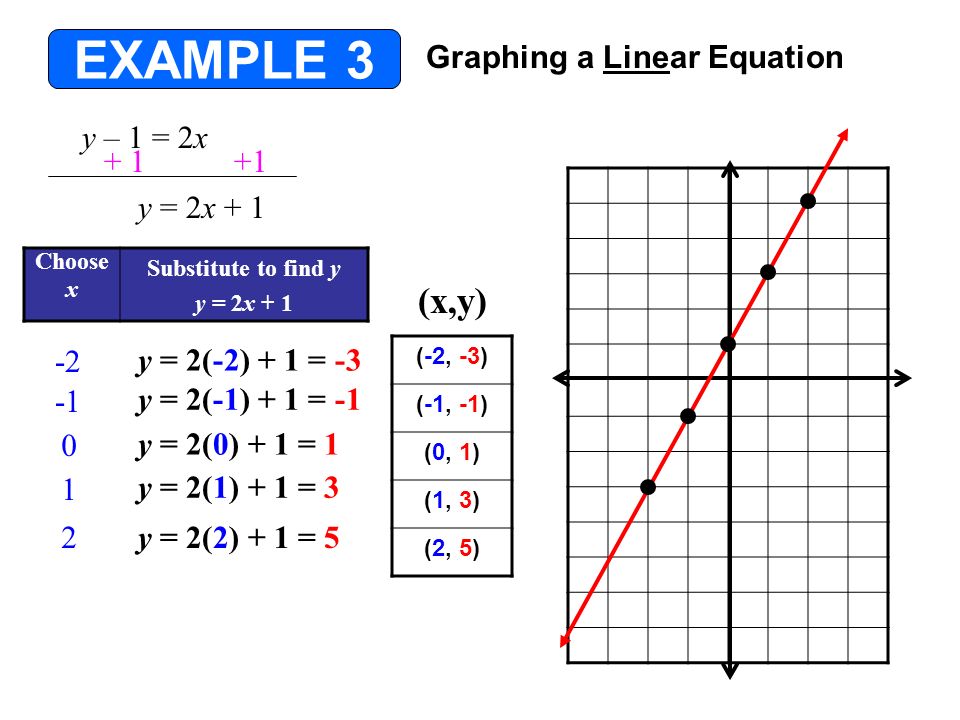



Graphing Linear Equations 4 2 Objective 1 Graph A Linear Equation Using A Table Or A List Of Values Objective 2 Graph Horizontal Or Vertical Lines Ppt Download




Solve System Of Algebraic Equations Matlab Simulink




Solve The Following Simultaneous Equation Using Cramer S Rule 2x 3y 2 X Y 2 1 2 Brainly In




Omtex Classes 3x Y 2 2x Y 3 Solve The Following Simultaneous Equations Graphically




Graph Graph Inequalities With Step By Step Math Problem Solver



Ex 3 6 1 I And Ii Solve 1 2x 1 3y 2 1 3x 1 2y



Grade 9 Math Quadratic Equations The Following Are Simultaneous Quadratic Equations I Ve Been Looking For A General Way For Solving Them But My Textbook Provides No Context And The Internet Hasn T Been




119 Ismsec1 Pdf Slope Square Root




Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook




Consider The Differential Equation Dy Dx Y 3 2 X Y 2 X 2 Statement 1 The Substitution Z Y 2 Transforms The Above Equation Into First Order Homogeneous Differential Equation Statement 2 The Solution Of This Differential Equation Is Y 2 E Y



Http Math Mit Edu Mckernan Teaching 10 11 Autumn 18 022 Model5 Pdf



Unit1 Vrs



0 件のコメント:
コメントを投稿